The Travelling knight
Can it move from A1 to H8, with a twist?
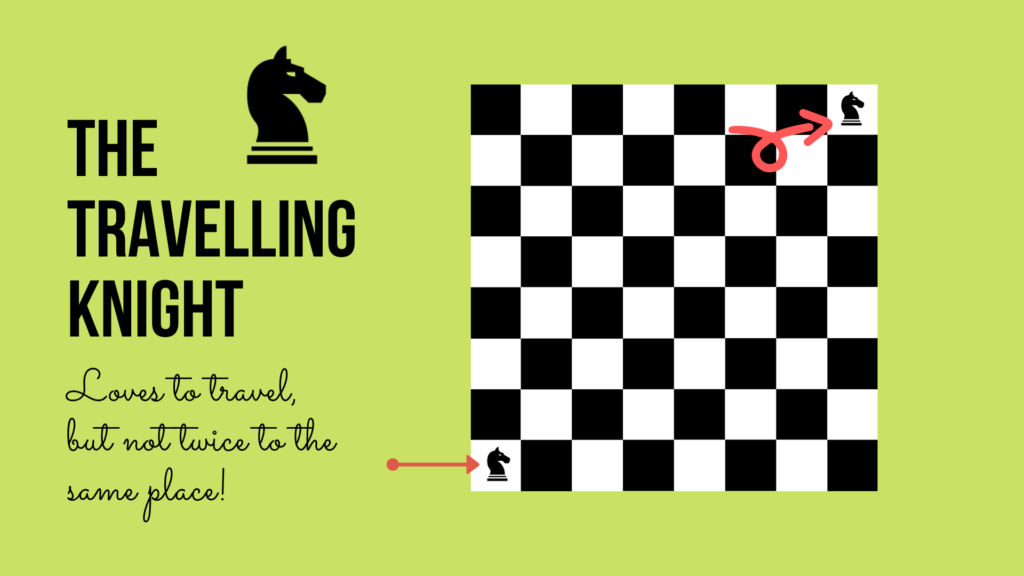
Yes, the knight loves to travel. But not twice to the same place!
The knight wants to move from position 1 (A1) to the final position (H8) as shown in the picture below.

However, there is 1 condition which it must follow on the way to its destination.
It should visit each of the remaining squares exactly once. So, it should visit every square on the chessboard but without any repetitions.
How would you do it? Is it even possible? Why?
Share your thoughts in the comments. A video explanation to the problem will be posted soon.
Note: If you are not into chess at all, you can check the problem below where you will also see a simple explanation of how a knight moves.
Check out the previous problem with a knight and 3×3 chessboard!
A1, C2, B4, C6, E5, F7, H8.
It is not possible. Since, a chess board has a total of 64 squares and knight is on a1 so remaining 63 squares are left to cover.Therefore , the knight has to move 63 times to cover remaining 63 squares. Now if a knight is on a white or black and moves even number of times then reaches a square which has same colour as the starting square. But 63 is odd so on the 62nd move the knight will be on a white square and all it’s surrounding black squares it can move to, should already be covered by… Read more »
I don’t think it’s possible.
What’s your explanation for this?
Same i don’t think it is possible