This post is for the students of Mathematical Curiosities, the Creative Math Live Online Program.
There are hundred bulbs in a room, which are all initially turned off. There are 100 people at the entrance of the room, who enter one by one. Each person changes the state of the all bulbs which are a multiple of that number. For example, 1st person turns on every bulb. The 2nd person toggles every 2nd bulb, and so on.
We already discussed in the class which bulbs will be finally switched on, after all 100 people have walked through. You can share your responses to these thinking questions and DIYs in the comments below, and start a discussion.
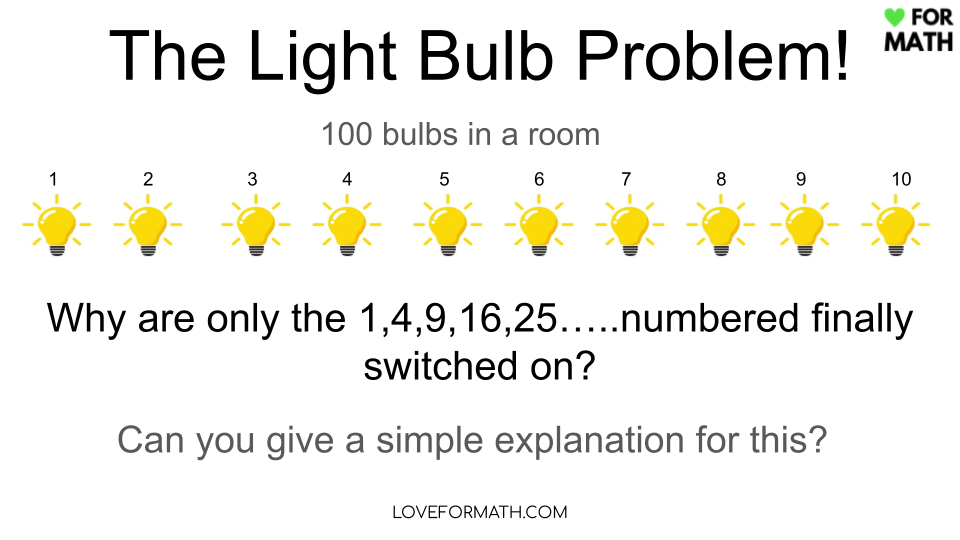


The answer to the 100 bulb problem is simply explained as follows :- Each Number can be represented as product of prime numbers raised to some powers. Say N = (a^x )X (b^y) X (c^z) where a,b,c are prime numbers Then total number of factors of N are (x+1)(y+1)(z+1) by basic counting. Now if this total number of factors will be odd then only Bulb will remain on otherwise switched off. Even factors imply that on->off->on->off ………. even number of toggles which results in the off state at the end. This is only true for perfect squares. Because, in that… Read more »